Finding Individual Molarity From a Solution

Molarity

Molarity is defined as the moles of a solute per volume of total solution.

Learning Objectives

Calculating solution concentrations using Molarity.

Key Takeaways

Key Points

• Molarity (M) indicates the number of moles of solute per liter of solution (moles/Liter) and is one of the most common units used to measure the concentration of a solution.
• Molarity can be used to calculate the volume of solvent or the amount of solute.
• The relationship between two solutions with the same amount of moles of solute can be represented by the formula c₁V₁ = c₂V₂, where c is concentration and V is volume.

Key Terms

• dilution: The process by which a solution is made less concentrated via addition of more solvent.
• SI unit: The modern form of the metric system used extensively in the sciences (abbreviated SI from French: Système International d'Unités).
• molarity: The concentration of a substance in solution, expressed as the number moles of solute per liter of solution.
• concentration: The relative amount of solute in a solution.

In chemistry, concentration of a solution is often measured in molarity (M), which is the number of moles of solute per liter of solution. This molar concentration (c_i) is calculated by dividing the moles of solute (n_i ) by the total volume (V) of the:

[latex]\text{c}_\text{i}=\frac{\text{n}_\text{i}}{\text{V}}[/latex]

The SI unit for molar concentration is mol/m³. However, mol/L is a more common unit for molarity. A solution that contains 1 mole of solute per 1 liter of solution (1 mol/L) is called "one Molar" or 1 M. The unit mol/L can be converted to mol/m³ using the following equation:

1 mol/L = 1 mol/dm³ = 1 mol dm⁻³ = 1 M = 1000 mol/m³

Calculating Molarity

To calculate the molarity of a solution, the number of moles of solute must be divided by the total liters of solution produced. If the amount of solute is given in grams, we must first calculate the number of moles of solute using the solute's molar mass, then calculate the molarity using the number of moles and total volume.

Calculating Molarity Given Moles and Volume

If there are 10.0 grams of NaCl (the solute) dissolved in water (the solvent) to produce 2.0 L of solution, what is the molarity of this solution?

First, we must convert the mass of NaCl in grams into moles. We do this by dividing by the molecular weight of NaCl (58.4 g/mole).

[latex]10.0 \text{ grams NaCl} \times \frac{\text{1 mole}}{58.4 \text {g/mole}} = 0.17 \text{ moles NaCl}[/latex]

Then, we divide the number of moles by the total solution volume to get concentration.

[latex]\text{c}_\text{i}=\frac{\text{n}_\text{i}}{\text{V}}[/latex]

[latex]\text{c}_\text{i}=\frac{0.17 \text{ moles NaCl}}{2 \text{ liters solution}}[/latex]

[latex]\text{c}_\text{i} = 0.1 \text{ M}[/latex]

The NaCl solution is a 0.1 M solution.

Calculating Moles Given Molarity

To calculate the number of moles in a solution given the molarity, we multiply the molarity by total volume of the solution in liters.

How many moles of potassium chloride (KCl) are in 4.0 L of a 0.65 M solution?

[latex]\text{c}_{\text{i}}=\frac{\text{n}_{\text{i}}}{\text{V}}[/latex]

[latex]0.65 \text{ M} = \frac{\text{n}_\text{i}}{4.0 \text{ L}}[/latex]

[latex]\text{n}_\text{i} = (0.65 \text{ M})(4.0 \text{ L}) = 2.6 \text{ moles KCl}[/latex]

There are 2.6 moles of KCl in a 0.65 M solution that occupies 4.0 L.

Calculating Volume Given Molarity and Moles

We can also calculate the volume required to meet a specific mass in grams given the molarity of the solution. This is useful with particular solutes that cannot be easily massed with a balance. For example, diborane (B₂H₆) is a useful reactant in organic synthesis, but is also highly toxic and flammable. Diborane is safer to use and transport if dissolved in tetrahydrofuran (THF).

How many milliliters of a 3.0 M solution of BH3-THF are required to receive 4.0 g of BH3?

First we must convert grams of BH₃ to moles by dividing the mass by the molecular weight.

[latex]\frac{4.0 \text{ g }\text{BH}_3 }{13.84 \text{g/mole }\text{BH}_3} = 0.29 \text{ moles }\text{BH}_3[/latex]

Once we know we need to achieve 0.29 moles of BH_3, we can use this and the given molarity (3.0 M) to calculate the volume needed to reach 4.0 g.

[latex]\text{c}_{\text{i}}=\frac{\text{n}_{\text{i}}}{\text{V}}[/latex]

[latex]3.0 \text{ M} = \frac{0.29 \text{moles BH}_3} {\text{V}}[/latex]

[latex]\text{V} = 0.1 \text{L}[/latex]

Now that we know that there are 4.0 g of BH₃ present in 0.1 L, we know that we need 100 mL of solution to obtain 4.0 g of BH₃.

Dilution

Dilution is the process of reducing the concentration of a solute in a solution, usually by adding more solvent. This relationship is represented by the equation c₁V₁ = c₂V₂, where c₁ and c₂ are the initial and final concentrations, and V₁ and V₂ are the initial and final volumes of the solution.

Example 1

A scientist has a 5.0 M solution of hydrochloric acid (HCl) and his new experiment requires 150.0 mL of 2.0 M HCl. How much water and how much 5.0 M HCl should the scientist use to make 150.0 mL of 2.0 M HCl?

c₁V₁ = c₂V₂

c₁ and V₁ are the concentration and the volume of the starting solution, which is the 5.0 M HCl. c₂ and V₂ are the concentration and the volume of the desired solution, or 150.0 mL of the 2.0 M HCl solution. The volume does not need to be converted to liters yet because both sides of the equation use mL. Therefore:

[latex](5.0 \text{ M HCl})(\text{V}_1) = (2.0 \text{ M HCl})(150.0 \text{ mL})[/latex]

V₁ = 60.0 mL of 5.0 M HCl

If 60.0 mL of 5.0 M HCl is used to make the desired solution, the amount of water needed to properly dilute the solution to the correct molarity and volume can be calculated:

150.0 mL – 60.0 mL = 90.0 mL

In order for the scientist to make 150.0 mL of 2.0 M HCl, he will need 60.0 mL of 5.0 M HCl and 90.0 mL of water.

Example 2

Water was added to 25 mL of a stock solution of 5.0 M HBr until the total volume of the solution was 2.5 L. What is the molarity of the new solution?

We are given the following: c₁= 5.o M, V₁= 0.025 L, V₂= 2.50 L. We are asked to find c₂, which is the molarity of the diluted solution.

(5.0 M)(0.025 L) = c₂ (2.50 L)

[latex]\text{c}_2 = \frac{(5.0 \text{M})(0.025 \text{L}) }{2.50 \text{L}} =0.05 \text{M}[/latex]

Notice that all of the units for volume have been converted to liters. We calculate that we will have a 0.05 M solution, which is consistent with our expectations considering we diluted 25 mL of a concentrated solution to 2500 mL.

Molality

Molality is a property of a solution that indicates the moles of solute per kilogram of solvent.

Learning Objectives

Calculate the molality of a solution and explain how it is a colligative property

Key Takeaways

Key Points

• Molality is a property of a solution and is defined as the number of moles of solute per kilogram of solvent.
• The SI unit for molality is mol/kg. A solution with a molality of 3 mol/kg is often described as "3 molal" or "3 m." However, following the SI system of units, mol/kg or a related SI unit is now preferred.
• Since the volume of a solution is dependent on ambient temperature and pressure, mass can be more relevant for measuring solutions. In these cases, molality (not molarity ) is the appropriate measurement.

Key Terms

• molality: The concentration of a substance in solution, expressed as the number of moles of solute per kilogram of solvent.
• colligative property: A property of solutions that depends on the ratio of the number of solute particles to the number of solvent molecules in a solution, and not on the type of chemical species present.
• intensive property: A property of matter that does not depend on the amount of matter.

Measurements of Mass (Molality) vs. Volume (Molarity)

Molality is an intensive property of solutions, and it is calculated as the moles of a solute divided by the kilograms of the solvent. Unlike molarity, which depends on the volume of the solution, molality depends only on the mass of the solvent. Since volume is subject to variation due to temperature and pressure, molarity also varies by temperature and pressure. In some cases, using weight is an advantage because mass does not vary with ambient conditions. For example, molality is used when working with a range of temperatures.

Defining Molality

The molality, b (or m), of a solution is defined as the amount of substance of solute in moles, n_solute, divided by the mass in kg of the solvent,m_solvent:

[latex]\text{bM}_{\text{solute}}=\frac{\text{n}_{\text{solute}}}{\text{m}_{\text{solvent}}}[/latex]

Molality is based on mass, so it can easily be converted into a mass ratio, denoted by w:

[latex]\text{bM}_{\text{solute}}=\frac{\text{n}_{\text{solute}}}{\text{m}_{\text{solvent}}} = \frac{\text{w}_{\text{solute}}}{\text{w}_{\text{solvent}}}[/latex]

Compared to molar concentration or mass concentration, the preparation of a solution of a given molality is easy because it requires only a good scale; both solvent and solute are massed, rather than measured by volume. In many weak aqueous solutions, the molarity and molality are similar because one kilogram of water (the solvent) occupies one liter of volume at room temperature, and the small amount of solute has little effect on the volume of the solvent.

A salt water solution: Table salt readily dissolves in water to form a solution. If the masses of the salt and of the water are known, the molality can be determined.

Units

The SI unit for molality is mol/kg, or moles solute per kg of solvent. A solution with a molality of 1 mol/kg is often described as "1 molal" or "1 m." However, following the SI system of units, the National Institute of Standards and Technology, which is the United States' authority on measurement, considers the term "molal" and the unit symbol "m" to be obsolete, and suggests using mol/kg or another related SI unit instead.

Calculating Molality

It is easy to calculate molality if we know the mass of solute and solvent in a solution. Molality is an intensive property, and is therefore independent of the amount being measured. This is true for all homogeneous solution concentrations, regardless of if we examine a 1.0 L or 10.0 L sample of the same solution. The concentration, or molality, remains constant.

Calculating Molality Given Mass

If we mass 5.36 g of KCl and dissolve this solid in 56 mL of water, what is the molality of the solution? Remember that molality is moles of solute/kg per solvent. KCl is our solute, while water is our solvent. We will first need to calculate the amount of moles present in 5.36 g of KCl:

[latex]\text{ moles KCl} = 5.36 \text{g} \times (\frac{1 \text{ moles}}{74.5 \text{g}}) = 0.0719 \text{ moles KCl}[/latex]

We also need to convert the the 56.0 mL of water to its equivalent mass in grams by using the known density of water (1.0 g/mL):

[latex]56.0\ \text{mL} \times (\frac{1.0 \text{g}}{\text{mL}}) = 56.0\ \text{g}[/latex]

56.0 g of water is equivalent to 0.056 kg of water. With this information, we can divide the moles of solute by the kg of solvent to find the molality of the solution:

[latex]\text{ molality} = (\frac {\text{ moles}}{\text{kg solvent}}) = (\frac {0.0719 \text{ moles KCl}}{0.056\text{ kg water}})= 1.3\ \text{m}[/latex]

The molality of our KCl and water solution is 1.3 m. Since the solution is very dilute, the molality is almost identical to the molarity of the solution, which is 1.3 M.

Calculating Mass Given Molality

We can also use molality to find the amount of a substance in a solution. For example, how much acetic acid, in mL, is needed to make a 3.0 m solution containing 25.0 g of KCN?

First, we must convert the sample of KCN from grams to moles:

[latex]\text{ moles KCN} = 25.0 \text{g} \times (\frac {1 \text{ moles}}{65.1 \text{g}}) = 0.38 \text{ moles}[/latex]

The moles of KCN can then be used to find the kg of acetic acid. We multiply the moles by the reciprocal of the given molality (3.0 moles/kg) so that our units appropriately cancel. The result is the desired mass of acetic acid that we need to make our 3 m solution:

[latex]0.38 \text{ moles KCl} \times (\frac {\text{ kg acetic acid}}{3.0 \text{ moles KCl}}) = 0.12\text{ kg acetic acid}[/latex]

Once we have the mass of acetic acid in kg, we convert from kg to grams: 0.12 kg is equal to 120 g. Next, we use the density of acetic acid (1.05 g/mL at 20 ^oC) to convert to the requested volume in mL. We must multiply by the reciprocal of the density to accomplish this:

[latex]120.0 \text{ g acetic acid} \times (\frac {\text{mL}}{1.05 \text{g}}) = 114.0 \text{ mL acetic acid}[/latex]

Therefore, we require 114 mL of acetic acid to make a 3.0 m solution that contains 25.0 g of KCN.

Molarity vs. molality: In this lesson, you will learn how molarity and molality differ.

Mole Fraction and Mole Percent

Mole fraction is the number of molecules of a given component in a mixture divided by the total number of moles in the mixture.

Learning Objectives

Calculate the mole fraction and mole percent for a given concentration of mixture

Key Takeaways

Key Points

• Mole fraction describes the number of molecules (or moles) of one component divided by total the number of molecules (or moles) in the mixture.
• Mole fraction is useful when two reactive components are mixed together, as the ratio of the two components is known if the mole fraction of each is known.
• Multiplying mole fraction by 100 gives mole percent, which describes the same thing as mole fraction, just in a different form. Mole fractions can be generated from various concentrations including molality, molarity and mass percent compositions.

Key Terms

• mole: The SI base unit for the amount of a substance; the amount of substance that contains as many elementary entities as there are atoms in 0.012 kg of carbon-12.
• mole fraction: The ratio of the number of moles of one component in a mixture to the total number of moles.

Mole Fraction

In chemistry, the mole fraction, x_i , is defined as the amount of moles of a constituent, n_i , divided by the total amount of moles of all constituents in a mixture, n_tot :

[latex]\text{x}_{\text{i}}=\frac{\text{n}_{\text{i}}}{\text{n}_{\text{tot}}}[/latex]

Mole fractions are dimensionless, and the sum of all mole fractions in a given mixture is always equal to 1.

Properties of the Mole Fraction

The mole fraction is used very frequently in the construction of phase diagrams. It has a number of advantages:

• It is not temperature dependent, as opposed to molar concentration, and does not require knowledge of the densities of the phase(s) involved.
• A mixture of known mole fractions can be prepared by weighing the appropriate masses of the constituents.
• The measure is symmetric; in the mole fractions x=0.1 and x=0.9, the roles of ' solvent ' and ' solute ' are reversible.
• In a mixture of ideal gases, the mole fraction can be expressed as the ratio of partial pressure to total pressure of the mixture.

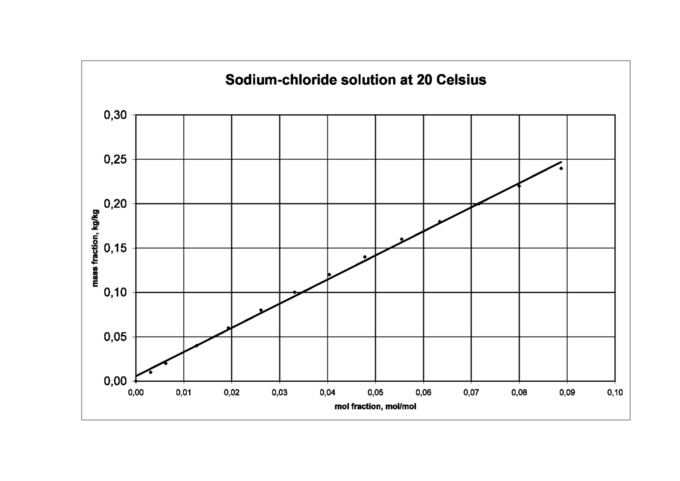
Mole fraction in a sodium chloride solution: Mole fraction increases proportionally to mass fraction in a solution of sodium chloride.

Mole Percent

Multiplying the mole fraction by 100 gives the mole percentage, also referred as amount/amount percent (abbreviated as n/n%). For general chemistry, all the mole percents of a mixture add up to 100 mole percent. We can easily convert mole percent back to mole fraction by dividing by 100. So, a mole fraction of 0.60 is equal to a mole percent of 60.0%.

Calculations with Mole fraction and Mole Percent

Mole Fraction in Mixtures

A mixture of gases was formed by combining 6.3 moles of O₂ and 5.6 moles of N₂. What is the mole fraction of nitrogen in the mixture?

First, we must find the total number of moles with n_total = n_N2 + n_O2.

[latex]\text{n}_{\text{total}}=6.3\ \text{moles}+5.6 \ \text{moles} = 11.9\ \text{moles}[/latex].

Next we must divide the moles of N₂ by the total number of moles:

[latex]x (\text{mole fraction}) = (\frac {\text{ moles } \text{N}_2}{\text{ moles } \text{N}_2 + \text{ moles } O_2})= (\frac {5.6 \text { moles}}{11.9 \text{ moles}})= 0.47[/latex]

The mole fraction of nitrogen in the mixture is 0.47.

Mole Fraction in Solutions

Mole fraction can also be applied in the case of solutions. For example, 0.100 moles of NaCl are dissolved in 100.0 mL of water. What is the mole fraction of NaCl?

We are given the number of moles of NaCl, but the volume of water. First, we convert this volume to a mass by using the density of water (1.00 g/mL), and then we convert this mass to moles of water:

[latex]100\ \text{mL}\ H_2O \times (\frac {1.0\text{g}}{1\text{mL}})= 100.0\ \text{g}\ \text{H}_2\text{O} \times (\frac {1 \text{ moles}}{18.0 g}) = 5.55 \text{ moles } \text{H}_2\text{O}[/latex]

With this information we can find the total number of moles present: 5.55 + 0.100 = 5.65 moles. If we divide the moles of NaCl by the total number of moles, we find the mole fraction of this component:

[latex]\text{x} = (\frac {0.100 \text {moles}}{5.65 \text {moles}}) = 0.0176[/latex]

We find that the mole fraction of NaCl is 0.0176.

Mole Fraction with Multi-Component Mixtures

Mole fractions can also be found for mixtures that are formed from multiple components. These are treated no differently than before; again, the total mole fraction of the mixture must be equal to 1.

For example, a solution is formed by mixing 10.0 g of pentane (C₅H₁₂), 10.0 g of hexane (C₆H₁₄) and 10.0 g of benzene (C₆H₆). What is the mole fraction of hexane in this mixture?

We must first find the number of moles present in 10.0 g of each component, given their chemical formulas and molecular weights. The number of moles for each is found by dividing its mass by its respective molecular weight. We find that there are 0.138 moles of pentane, 0.116 moles of hexane, and 0.128 moles of benzene.

We can find the total number of moles by taking the sum of all the moles: 0.138+0.116+0.128 = 0.382 total moles. If we divide moles of hexane by the total moles, we calculate the mole fraction:

[latex]\text{x} = (\frac {0.116 \text{ moles}}{0.382 \text{ moles}}) = 0.303[/latex]

The mole fraction for hexane is 0.303.

Mole Fraction from Molality

Mole fraction can also be calculated from molality. If we have a 1.62 m solution of table sugar (C₆H₁₂O₆) in water, what is the mole fraction of the table sugar?

Since we are given molality, we can convert it to the equivalent mole fraction, which is already a mass ratio; remember that molality = moles solute/kg solvent. Given the definition of molality, we know that we have a solution with 1.62 moles of sugar and 1.00 kg (1000 g) of water. Since we know the number of moles of sugar, we need to find the moles of water using its molecular weight:

[latex]1000\ \text{g}\ \text{H}_2\text{O} \times (\frac {1\ \text{mole}}{18.0\ \text{g}}) = 55.5 \text{ moles }\text{H}_2\text{O}[/latex]

The total number of moles is the sum of the moles of water and sugar, or 57.1 moles total of solution. We can now find the mole fraction of the sugar:

[latex]\text{x} = (\frac {1.62 \text{ moles sugar}}{57.1 \text{ moles solution}})= 0.0284 [/latex]

With the mole fraction of 0.0284, we see that we have a 2.84% solution of sugar in water.

Mole Fraction from Mass Percent

The mole fraction can also be calculated from a mass percent. What is the mole fraction of cinnamic acid that has a mass percent of 50.00% urea in cinnamic acid? The molecular weight of urea is 60.16 g/mol and the molecular weight of cinnamic acid is 148.16 g/mol.

First, we assume a total mass of 100.0 g, although any mass could be assumed. This means that we have 50.0 g of urea and 50.0 g of cinnamic acid. We can then calculate the moles present by dividing each by its molecular weight. We have 0.833 moles urea and 0.388 moles cinnamic acid, so we have 1.22 moles total.

To find the mole fraction, we divide the moles of cinnamic acid by total number of moles:

[latex]\text{x} = (\frac {.388 \text{ moles cinnamic acid}}{1.22 \text{ moles solution}})= 0.318[/latex]

The mole fraction for cinnamic acid is 0.318.

Finding Individual Molarity From a Solution

Source: https://courses.lumenlearning.com/boundless-chemistry/chapter/concentration-units/

Share :